|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
https://www.quantamagazine.org/quantum-tunnel-shows-particles-can-break-the-speed-of-light-20201020/?utm_source=Nature+Briefing&utm_campaign=b3fb4910e9-briefing-dy-20201022&utm_medium=email&utm_term=0_c9dfd39373-b3fb4910e9-43398473 |
|
|
|
Quantum Tunnels Show How
Particles Can Break the Speed of Light |
|
|
Recent experiments show that
particles should be able to go faster than light when they quantum
mechanically “tunnel” through walls. |
|
|
|
|
|
18 |
|
|
READ LATER |
|
|
|
|
|
The bizarre rules of quantum mechanics allow a particle to
occasionally pass through a seemingly impenetrable barrier. |
|
|
|
|
|
Maylee for Quanta Magazine |
|
|
|
|
|
|
|
|
|
|
|
Natalie
Wolchover |
|
|
Senior
Writer/Editor |
|
|
|
|
|
|
|
|
|
October 20, 2020 |
|
|
|
|
|
|
|
|
|
VIEW PDF/PRINT MODE |
|
|
|
|
|
Experimental
PhysicsPhysicsQuantum PhysicsAll topics |
|
|
|
|
|
No sooner had the radical
equations of quantum mechanics been discovered than physicists identified one
of the strangest phenomena the theory allows. |
|
|
|
|
|
“Quantum
tunneling” shows how profoundly particles such as electrons differ from
bigger things. Throw a ball at the wall and it bounces backward; let it roll
to the bottom of a valley and it stays there. But a particle will
occasionally hop through the wall. It has a chance of “slipping through the
mountain and escaping from the valley,” as two physicists wrote
in Nature in 1928, in one of the earliest descriptions of
tunneling. |
|
|
|
|
|
Physicists quickly saw that
particles’ ability to tunnel through barriers solved many mysteries. It
explained various chemical bonds and radioactive decays and how hydrogen
nuclei in the sun are able to overcome their mutual repulsion and fuse,
producing sunlight. |
|
|
|
|
|
But physicists became curious
— mildly at first, then morbidly so. How long, they wondered, does it take
for a particle to tunnel through a barrier? |
|
|
|
|
|
The trouble was that the
answer didn’t make sense. |
|
|
|
|
|
The first tentative
calculation of tunneling time appeared in print in 1932. Even
earlier stabs might have been made in private, but “when you get an answer
you can’t make sense of, you don’t publish it,” noted Aephraim
Steinberg, a physicist at the University of Toronto. |
|
|
|
|
|
It
wasn’t until 1962 that a semiconductor engineer at Texas Instruments named
Thomas Hartman wrote a paper that explicitly embraced the shocking
implications of the math. |
|
|
|
|
|
Hartman found that a barrier
seemed to act as a shortcut. When a particle tunnels, the trip takes less
time than if the barrier weren’t there. Even more astonishing, he calculated
that thickening a barrier hardly increases the time it takes for a particle
to tunnel across it. This means that with a sufficiently thick barrier,
particles could hop from one side to the other faster than light traveling
the same distance through empty space. |
|
|
|
|
|
In short, quantum tunneling
seemed to allow faster-than-light travel, a supposed physical impossibility. |
|
|
|
|
|
“After the Hartman effect,
that’s when people started to worry,” said Steinberg. |
|
|
|
|
|
The
discussion spiraled for decades, in part because the tunneling-time question
seemed to scratch at some of the most enigmatic aspects of quantum mechanics.
“It’s part of the general problem of what is time, and how do we measure time
in quantum mechanics, and what is its meaning,” said Eli Pollak, a
theoretical physicist at the Weizmann Institute of Science in Israel.
Physicists eventually derived at least 10 alternative mathematical
expressions for tunneling time, each reflecting a different perspective on
the tunneling process. None settled the issue. |
|
|
|
|
|
|
But the tunneling-time
question is making a comeback, fueled by a series of virtuoso experiments
that have precisely measured tunneling time in the lab. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Aephraim Steinberg, a
physicist at the University of Toronto, has pursued the tunneling-time
question for decades. |
|
|
Matthew Ross |
|
|
|
|
|
In
the most highly praised measurement yet, reported in Nature in
July, Steinberg’s group in Toronto used what’s called the Larmor clock method
to gauge how long rubidium atoms took to tunnel through a repulsive laser
field. |
|
|
|
|
|
“The Larmor clock is the best
and most intuitive way to measure tunneling time, and the experiment was the
first to very nicely measure it,” said Igor Litvinyuk, a physicist at
Griffith University in Australia who reported a different measurement of
tunneling time in Nature last
year. |
|
|
|
|
|
Luiz Manzoni, a theoretical physicist at Concordia College
in Minnesota, also finds the Larmor clock measurement convincing. “What they
measure is really the tunneling time,” he said. |
|
|
|
|
|
The recent experiments are
bringing new attention to an unresolved issue. In the six decades since
Hartman’s paper, no matter how carefully physicists have redefined tunneling
time or how precisely they’ve measured it in the lab, they’ve found that
quantum tunneling invariably exhibits the Hartman effect. Tunneling seems to
be incurably, robustly superluminal. |
|
|
|
|
|
“How is it possible for [a
tunneling particle] to travel faster than light?” Litvinyuk said. “It was
purely theoretical until the measurements were made.” |
|
|
|
|
|
What Time? |
|
|
|
|
|
Tunneling time is hard to pin
down because reality itself is. |
|
|
|
|
|
At the macroscopic scale, how
long an object takes to go from A to B is simply the distance divided by the
object’s speed. But quantum theory teaches us that precise knowledge of both
distance and speed is forbidden. |
|
|
|
|
|
In quantum theory, a particle
has a range of possible locations and speeds. From among these options,
definite properties somehow crystallize at the moment of measurement. How
this happens is one of the deepest questions. |
|
|
|
|
|
The upshot is that until a
particle strikes a detector, it’s everywhere and nowhere in particular. This
makes it really hard to say how long the particle previously spent somewhere,
such as inside a barrier. “You cannot say what time it spends there,” Litvinyuk
said, “because it can be simultaneously two places at the same time.” |
|
|
|
|
|
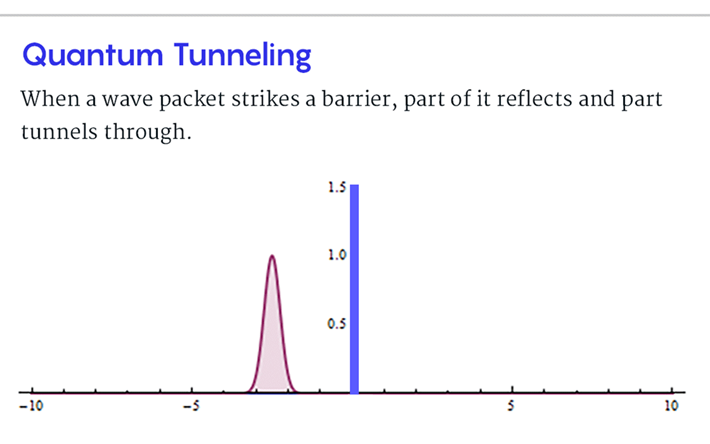
To understand the problem in
the context of tunneling, picture a bell curve representing the possible
locations of a particle. This bell curve, called a wave packet, is centered
at position A. Now picture the wave packet traveling, tsunami-like, toward a
barrier. The equations of quantum mechanics describe how the wave packet
splits in two upon hitting the obstacle. Most of it reflects, heading back
toward A. But a smaller peak of probability slips through the barrier and
keeps going toward B. Thus the particle has a chance of registering in a
detector there. |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Yuvalr |
|
|
|
|
|
But when a particle arrives at
B, what can be said about its journey, or its time in the barrier? Before it
suddenly showed up, the particle was a two-part probability wave — both
reflected and transmitted. It both entered the barrier and didn’t. The meaning
of “tunneling time” becomes unclear. |
|
|
|
|
|
And yet any particle that
starts at A and ends at B undeniably interacts with the barrier in between,
and this interaction “is something in time,” as Pollak put it. The question
is, what time is that? |
|
|
|
|
|
Steinberg, who has had “a
seeming obsession” with the tunneling-time question since he was a graduate
student in the 1990s, explained that the trouble stems from the peculiar
nature of time. Objects have certain characteristics, like mass or location.
But they don’t have an intrinsic “time” that we can measure directly. “I can
ask you, ‘What is the position of the baseball?’ but it makes no sense
to ask, ‘What is the time of the baseball?’” Steinberg said. “The time
is not a property any particle possesses.” Instead, we track other changes in
the world, such as ticks of clocks (which are ultimately changes in
position), and call these increments of time. |
|
|
|
But in the tunneling scenario,
there’s no clock inside the particle itself. So what changes should be
tracked? Physicists have found no end of possible proxies for tunneling time. |
|
|
|
Tunneling Times |
|
|
|
Hartman (and LeRoy Archibald
MacColl before him in 1932) took the simplest approach to gauging how long
tunneling takes. Hartman calculated the difference in the most likely arrival
time of a particle traveling from A to B in free space versus a particle that
has to cross a barrier. He did this by considering how the barrier shifts the
position of the peak of the transmitted wave packet. |
|
|
|
But this approach has a
problem, aside from its weird suggestion that barriers speed particles up.
You can’t simply compare the initial and final peaks of a particle’s wave
packet. Clocking the difference between a particle’s most likely departure
time (when the peak of the bell curve is located at A) and its most likely
arrival time (when the peak reaches B) doesn’t tell you any individual
particle’s time of flight, because a particle detected at B didn’t
necessarily start at A. It was anywhere and everywhere in the initial
probability distribution, including its front tail, which was much closer to
the barrier. This gave it a chance to reach B quickly. |
|
|
|
It’s part of the general
problem of what is time, and how do we measure time in quantum mechanics, and
what is its meaning.” |
|
|
|
Eli Pollak |
|
|
|
Since particles’ exact
trajectories are unknowable, researchers sought a more probabilistic
approach. They considered the fact that after a wave packet hits a barrier,
at each instant there’s some probability that the particle is inside the
barrier (and some probability that it’s not). Physicists then sum up the
probabilities at every instant to derive the average tunneling time. |
|
|
|
As for how to measure the
probabilities, various thought experiments were conceived starting in the
late 1960s in which “clocks” could be attached to the particles themselves.
If each particle’s clock only ticks while it’s in the barrier, and you read
the clocks of many transmitted particles, they’ll show a range of different
times. But the average gives the tunneling time. |
|
|
|
|
All of this was easier said than done, of course. “They were
just coming up with crazy ideas of how to measure this time and thought it
would never happen,” said Ramón Ramos, the lead author of the
recent Nature paper. “Now the science has advanced, and we were
happy to make this experiment real.” |
|
|
|
|
|
Embedded Clocks |
|
|
|
|
|
Although physicists have
gauged tunneling times since the 1980s, the recent rise of ultraprecise
measurements began in 2014 in Ursula Keller’s lab at the Swiss Federal
Institute of Technology Zurich. Her team measured tunneling
time using what’s called an attoclock. In Keller’s attoclock, electrons
from helium atoms encounter a barrier, which rotates in place like the hands
of a clock. Electrons tunnel most often when the barrier is in a certain
orientation — call it noon on the attoclock. Then, when electrons emerge from
the barrier, they get kicked in a direction that depends on the barrier’s
alignment at that moment. To gauge the tunneling time, Keller’s team measured
the angular difference between noon, when most tunneling events began, and
the angle of most outgoing electrons. They measured a difference of 50
attoseconds, or billionths of a billionth of a second. |
|
|
|
|
|
Then in work reported in 2019,
Litvinyuk’s group improved on Keller’s attoclock experiment by switching from
helium to simpler hydrogen atoms. They measured an even shorter time of at
most two attoseconds, suggesting that tunneling happens almost instantaneously. |
|
|
|
|
|
But some experts have since concluded that the duration the
attoclock measures is not a good proxy for tunneling time. Manzoni, who
published an analysis of the measurement last year, said the
approach is flawed in a similar way to Hartman’s tunneling-time definition:
Electrons that tunnel out of the barrier almost instantly can be said, in
hindsight, to have had a head start. |
|
|
|
|
|
Meanwhile, Steinberg, Ramos
and their Toronto colleagues David Spierings and Isabelle Racicot pursued an
experiment that has been more convincing. |
|
|
|
|
|
This alternative approach
utilizes the fact that many particles possess an intrinsic magnetic property
called spin. Spin is like an arrow that is only ever measured pointing up or
down. But before a measurement, it can point in any direction. As the Irish
physicist Joseph Larmor discovered in 1897, the angle of the spin rotates, or
“precesses,” when the particle is in a magnetic field. The Toronto team used
this precession to act as the hands of a clock, called a Larmor clock. |
|
|
|
|
|
The researchers used a laser
beam as their barrier and turned on a magnetic field inside it. They then
prepared rubidium atoms with spins aligned in a particular direction, and
sent the atoms drifting toward the barrier. Next, they measured the spin of the
atoms that came out the other side. Measuring any individual atom’s spin
always returns an unilluminating answer of “up” or “down.” But do the
measurement over and over again, and the collected measurements will reveal
how much the angle of the spins precessed, on average, while the atoms were
inside the barrier — and thus how long they typically spent there. |
|
|
|
|
|
|
|
|
|
|
|
|
|
Samuel Velasco/Quanta Magazine |
|
|
|
|
|
The researchers reported that the rubidium atoms spent, on
average, 0.61 milliseconds inside the barrier, in line with Larmor clock
times theoretically predicted in the 1980s. That’s less time than the atoms
would have taken to travel through free space. Therefore, the calculations
indicate that if you made the barrier really thick, Steinberg said, the
speedup would let atoms tunnel from one side to the other faster than light. |
|
|
|
|
|
A Mystery, Not a Paradox |
|
|
|
|
|
In 1907, Albert Einstein realized that his brand-new theory
of relativity must render faster-than-light communication impossible. Imagine
two people, Alice and Bob, moving apart at high speed. Because of relativity,
their clocks tell different times. One consequence is that if Alice sends a
faster-than-light signal to Bob, who immediately sends a superluminal reply
to Alice, Bob’s reply could reach Alice before she sent her initial message.
“The achieved effect would precede the cause,” Einstein wrote. |
|
|
|
|
|
Experts generally feel
confident that tunneling doesn’t really break causality, but there’s no
consensus on the precise reasons why not. “I don’t feel like we have a
completely unified way of thinking about it,” Steinberg said. “There’s a
mystery there, not a paradox.” |
|
|
|
|
|
Tunneling “almost seems
weirder than entanglement.” |
|
|
|
|
|
Grace Field |
|
|
|
|
|
Some good guesses are wrong.
Manzoni, on hearing about the superluminal tunneling issue in the early
2000s, worked with a colleague to redo the calculations. They thought they
would see tunneling drop to subluminal speeds if they accounted for
relativistic effects (where time slows down for fast-moving particles). “To
our surprise, it was possible to have superluminal tunneling there too,”
Manzoni said. “In fact, the problem was even more drastic in relativistic
quantum mechanics.” |
|
|
|
|
|
Researchers stress that
superluminal tunneling is not a problem as long as it doesn’t allow
superluminal signaling. It’s similar in this way to the “spooky action at a
distance” that so bothered Einstein. Spooky action refers to the ability of
far-apart particles to be “entangled,” so that a measurement of one instantly
determines the properties of both. This instant connection between distant
particles doesn’t cause paradoxes because it can’t be used to signal from one
to the other. |
|
|
|
|
|
Considering the amount of hand-wringing over spooky action
at a distance, though, surprisingly little fuss has been made about
superluminal tunneling. “With tunneling, you’re not dealing with two systems
that are separate, whose states are linked in this spooky way,”
said Grace Field, who studies the tunneling-time issue at the University
of Cambridge. “You’re dealing with a single system that’s traveling through
space. In that way it almost seems weirder than entanglement.” |
|
|
|
|
|
In a paper published in the New Journal of
Physics in September, Pollak and two colleagues argued that superluminal
tunneling doesn’t allow superluminal signaling for a statistical reason: Even
though tunneling through an extremely thick barrier happens very fast, the
chance of a tunneling event happening through such a barrier is
extraordinarily low. A signaler would always prefer to send the signal
through free space. |
|
|
|
|
|
Why, though, couldn’t you
blast tons of particles at the ultra-thick barrier in the hopes that one will
make it through superluminally? Wouldn’t just one particle be enough to
convey your message and break physics? Steinberg, who agrees with the
statistical view of the situation, argues that a single tunneled particle
can’t convey information. A signal requires detail and structure, and any
attempt to send a detailed signal will always be faster sent through the air
than through an unreliable barrier. |
|
|
|
|
|
Pollak said these questions
are the subject of future study. “I believe the experiments of Steinberg are
going to be an impetus for more theory. Where that leads, I don’t know.” |
|
|
|
|
|
RELATED: |
|
|
|
|
|
|
Room-Temperature Superconductivity Achieved for the First
Time |
|
|
Does Time Really Flow? New Clues Come From a Century-Old
Approach to Math. |
|
|
Dark Matter Experiment Finds Unexplained Signal |
|
|
|
|
|
The pondering will occur
alongside more experiments, including the next on Steinberg’s list. By
localizing the magnetic field within different regions in the barrier, he and
his team plan to probe “not only how long the particle spends in the barrier,
but where within the barrier it spends that time,” he said. Theoretical
calculations predict that the rubidium atoms spend most of their time near
the barrier’s entrance and exit, but very little time in the middle. “It’s
kind of surprising and not intuitive at all,” Ramos said. |
|
|
|
|
|
By probing the average
experience of many tunneling particles, the researchers are painting a more
vivid picture of what goes on “inside the mountain” than the pioneers of
quantum mechanics ever expected a century ago. In Steinberg’s view, the
developments drive home the point that despite quantum mechanics’ strange
reputation, “when you see where a particle ends up, that does give you more
information about what it was doing before.” |
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|